Exercise 6.2 Class 10 Maths Chapter 6 Triangles Solution

Exercise 6.2 Class 10 Maths Chapter 6 Triangles Solution

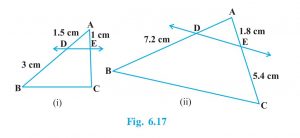
1. In Fig. 6.17, (i) and (ii), DE || BC. Find EC in (i) and AD in (ii).

Solution:

(i) Consider triangle ABC and ADE

∠ BAC = ∠  DAE

Corresponding angles in parallel lines are equal.

So, ∠ ABC =  ∠ ADE

∠ ACB = ∠ AED

Hence, Both triangles are equiangular. Now, As per Thale’s theorem, Equiangular triangles are similar triangles.

ΔABC ~ ΔADE

So, AB/AD = AC/AE = BC/DE

i.e. 4.5/1.5 = AC/1

So, AC= 3cm.

Hence, EC = AC – AE

= 2cm.

(ii) Similar to question (i)

ΔABC ~ ΔADE

i.e. AB/AD = AC/AE

AB/AD = 7.2/1.8

so, AB/AD = 4

i.e. AB = 4AD

=> AD + 7.2 = 4AD

=> 3AD= 7.2

Hence, AD = 2.4cm.

2. E and F are points on the sides PQ and PR respectively of a ∆ PQR. For each of the following cases, state whether EF || QR :
(i) PE = 3.9 cm, EQ = 3 cm, PF = 3.6 cm and FR = 2.4 cm
(ii) PE = 4 cm, QE = 4.5 cm, PF = 8 cm and RF = 9 cm
(iii) PQ = 1.28 cm, PR = 2.56 cm, PE = 0.18 cm and PF = 0.36 cm

Answer: Line EF and QR will be parallel if ∆ PQR ~ ∆ PEF.

(i) PE/PQ = 3.9/6.9 = 0.565; PF/PR = 3.6/7 = 0.514.

Here Ratio of sides of the triangle is not the same, so both the triangles will not be similar. Hence line EF will not be parallel to line QR.

(ii) The ratio of sides of triangles will be:

PE/PQ = 4/8.5 = 0.47; PF/PR = 8/17 = 0.47.

Here, both triangles are similar so EF || QR.

(iii) PE/PQ = 0.18/1.28 = 0.14; PF/PR = 0.36/2.56 = 0.14.

Here, both triangles are similar so EF || QR.

3. In Fig. 6.18, if LM || CB and LN || CD, prove that AM/AB =  AN/AD
Answer:

Here we need to prove that triangle ABC and ADC are similar.

Consider ∆ANL and ∆ADC

Since, LN || CD,

∠ANL = ∠ADC and ∠ALN = ∠ACD

So, ∆ANL ~ ∆ADC

So, AN/AD = AL/AC = LN/CD  (Finding i)

Similalrly,

∆AML ~ ∆ABC

So, AL/AC = AM/AB = LM/BC  (Finding ii)

In both the findings, AL/AC is common

So,

∆ANL ~ ∆ABC

∆AML ~ ∆ADC

Thus, ∆ABC~ ∆ADC

Hence, AM/AB =  AN/AD.

4. In Fig. 6.19, DE || AC and DF || AE. Prove that BF/FE =  BE/EC

Answer:

Given that, DE || AC. So, ∆ABC ~ ∆BDE ;

so, BE/BC = BD/BA = DE/AC.

DF || AE, So,  ∆ABE ~ ∆BDF

So, FE/EC = DF/AE = DE/AC.

We need to prove, BF/FE = BE/EC i.e. BF/BE = FE/EC

Now,

BF/BE = BD/BA (Since, ABE ~ ∆BDF)  (i)

DE/AC = BD/BA (SInce, ∆ABC ~ ∆BDE)  (ii)

From i and ii we get BF/BE = DE/AC.

5. In Fig. 6.20, DE || OQ and DF || OR. Show that EF || QR.

Answer:  Since DE || OQ,

PE/PQ = PD/PO = DE/OQ

Since DF || OR,

PD/PO = PF/PR = DF/OR

Since i and ii have PD/PO in common So,

PE/PQ = PF/PR  (i)

In triangle PEF and PQR, they have common angle PEF and PQR (ii)

From I and ii, ∆PEF ~ ∆PQR so, ∠PEF = PQR.

Hence, EF || QR.